Complete stepbystep answer We are given that x y z = 0 We will take z to other side and rewrite the equation as, x y = − z (1) Since, we want to find the value of x 3 y 3 z 3 and allSolution Given that 1 (1/2) 3 (3/2) 3 If x y z = 0, then x 3 y 3 z 3 = 3xyz x = 1, y = 1/2 and z = 3/2 x y z = 1 (1/2) (3/2) = 0 1 (1/2)3 (3/2)3 = 3 (1) (1/2) (3/2) = 9/4 Class 12 English Class 12 Accountancy Class 12 Economics Class 12 Computer Science (Python) Class 12 Physics Class 12 Chemistry Class 12 Biology Class 12 Physical

Pdf On Solving The Diophantine Equation 𝑥 𝑦 𝑧 𝑘 On A Vector Computer
X^3+y^3+z^3-3xyz all formula
X^3+y^3+z^3-3xyz all formula- If 3xyz=0 show that 27x 3 y 3 z 3 =9xyz Share with your friends 4 Follow 0 Moktak, added an answer, on Identity x 3 y 3 z 3 3xyz = (xyz) (x 2 y 2 z 22 Consider the surface defined by the equation x^3 y^3 z^3 =3xyz Use implicit differentiation to find partial z/ partial x Question 2 Consider the surface defined by the equation x^3 y^3




Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15
Given an integer $N > 0$ with unknown factorization, I would like to find nontrivial solutions $(X, Y, Z)$ to the congruence $X^3 Y^3 Z^3 3XYZ \equiv 1 \pmod{NIf x y z = 2, x 3 y 3 z 3 3xyz = 74, then (x 2 y 2 z 2) is equal to This question was previously asked in SSC CGL TierI Official Paper 18 (Held On Shift 3)Class 12 humanities 3 ( zx)3
Notice this cool factorization a^3b^3c^33abc=(abc)(a^2b^2c^2abbcac)=\frac{1}{2}(abc)((ab)^2(bc)^2(ac)^2) Apply it for a=x, b=y, c=1 to obtain\textbf{Hint} Note that x^3y^3z^33xyz=(xyz)(x^2y^2z^2xyyzzx)=\frac{1}{2}(xyz)(xy)^2(yz)^2(zx)^2=0 if and only if either xyz=0 or x=y,y=z and z=x Now count the ForExamples Using Factoring Formulas Example 1 Factorize the expression 8x 3 27 Solution To factorize 8x 3 27 We will use the a 3 b 3 formula (one of the special factoring formulas) to
If the polynomial k 2 x 3 − kx 2 3kx k is exactly divisible by (x3) then It seems more like this should be an inequality (xy)^3 (yz)3 (zx)^3 >= 3 (xy) (yz) (zx) But that is not the question set Please note that this is the first chapter and all thatThere are two formula of it 1 x^3 y^3 z^3 3xyz = (xyz) (x^2y^2z^2xyyzzx) 2 x^3 y^3 z^3 3xyz = (1/2) (xyz) {xy)^2(yz)^2(zx)^2}



Using Identities Prove That X 3 Y 3 Z 3 Or Equal To 3xyz Where X Y Z Are Positive Real Numbers Quora



Halp Mx1 Exam Tomorrow Bored Of Studies
(xyz)^3 put xy = a (az)^3= a^3 z^3 3az ( az) = (xy)^3 z^3 3 a^2 z 3a z^2 = x^3y^3 z^3 3 x^2 y 3 x y^2 3(xy)^2 z 3(xy) z^2 =x^3 y^3 z^3 3 x^2y 3xy^2 3 ( x^2 y^2If x y z = 6 and xy yz zx = 10, then the value of x3 y3 z3 3xyz is? What must be subtracted from 4x^42x^36x^22x6 so that the result is exactly divisible by 2x^2x1?



How To Prove That Math X Y Z 3 27xyz Geq0 Math Quora



If X Z 225 And Y 226 Then What Is The Value Of X Y Z 3xyz Quora
A 3 B x2 C y D z # B ∵ ∴ 3xyz = 3 x yIf x y z = 7, x2 y2 z2 = 85 and x3 y3 z3 = 913, then the value of \(\rm \sqrt3{xyz}\) is Explore Exams Practice Papers Test Series News Register Quantitative Aptitude;View Formulae MC ANSpdf from CAES 90 at The University of Hong Kong Which of the following is NOT a common factor of 3xyz and 9x2yz2?




Factorise 8x 3 Y 3 27z 3 18 Xyz




X 3 Y 3 Z 3 3xyz X Y Z X 2 Y 2 Z 2 Xy Yz Zx A 3 B 3 C 3 3abc A B C A 2 B 2 C 2 Ab Ca Youtube
1 Given V = (x^3)(y) yz c (z^2), find C such that V satisfies laplace equation 2 Le U = 3xyz y z^2 Show whether or not U satisfies laplace's equation Question 1 Given V = (x^3)(y) yz It is x3 y3 z3 −3xyz = x3 y3 3x2y 3xy2 z3 − 3xyz − 3x2y − 3xy2 = (x y)3 z3 − 3xy(x y z) = (x y z)((x y)2 z2 −(x y)z) −3xy(x y z) = (x yShow 2 more comments 0 (This is only a partial answer to the question) First, we have x 3 y 3 z 3 = x y z = 3 Assume that x, y, and z are all positive Note that, by the Arithmetic Mean




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes



If X Y Z 0 Show That X3 Y3 Z3 3xyz
Put u = x y, v = z in the formula x 3 y 3 z 33 x y z = x y z x y 2 z 2x y z3 x y x y z ⇒ x 3 y 3 z 33 x y z = x y z x y 2 z 2x zy z3 x y x y z ∵ (x y) 2 = (x 2 y 2 Find the value of (x3y3z3 3xyz), if x yz = 9 and xy yzzx = 26a)27b)52c)81d)576Correct answer is option 'A' Can you explain this answer?Let → a,→ b and → c be mutually perpendicular vectors of the same magnitudeIf a vector → x satisfies the equation Q Let f(x)= (x−3)(x2)(x6) (x1)(x−5) Find intervals, where f(x) is




Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Class 9th Ex 2 5 Question 12 Youtube




Factorization Of X3 Y3 Z3 3xyz Youtube
Factor(x^3y^3z^3 ) Natural Language; Conversely, given any nonzero $\alpha \in {\bf Q}(\omega)$ we solve these linear equations in $x,y,z\in\bf Q$ to obtain the general solution of $x^3 y^3 z^3 3xyz = 1$Share It On Facebook Twitter Email 1 Answer 0 votes answered




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




Showimage Php Formula B5dad96cb1f3c6313bc6 Png D 0
Share It On Facebook Twitter Email 1 Answer 0 votesLike Dislike Share Save NumberX 148K subscribers x^3y^3z^33xyz= (xyz) (x^2y^2z^2xyyzzx) a^3b^3c^33abc= (abc) (a^2b^2c^2abbcca) a^3b^3c^3Solution Verified by Toppr x 3y 3z 3−3xyz=(xyz)(x 2y 2z 2−xy−yz−zx) First take LHS (xyz)(x 2y 2z 2−xy−yz−zx) To multiply two polynomials, we multiply each monomial of one




If U Log X 3 Y 3 Z 3 3xyz And X Y Z 2u K X Y Z 2 Then K
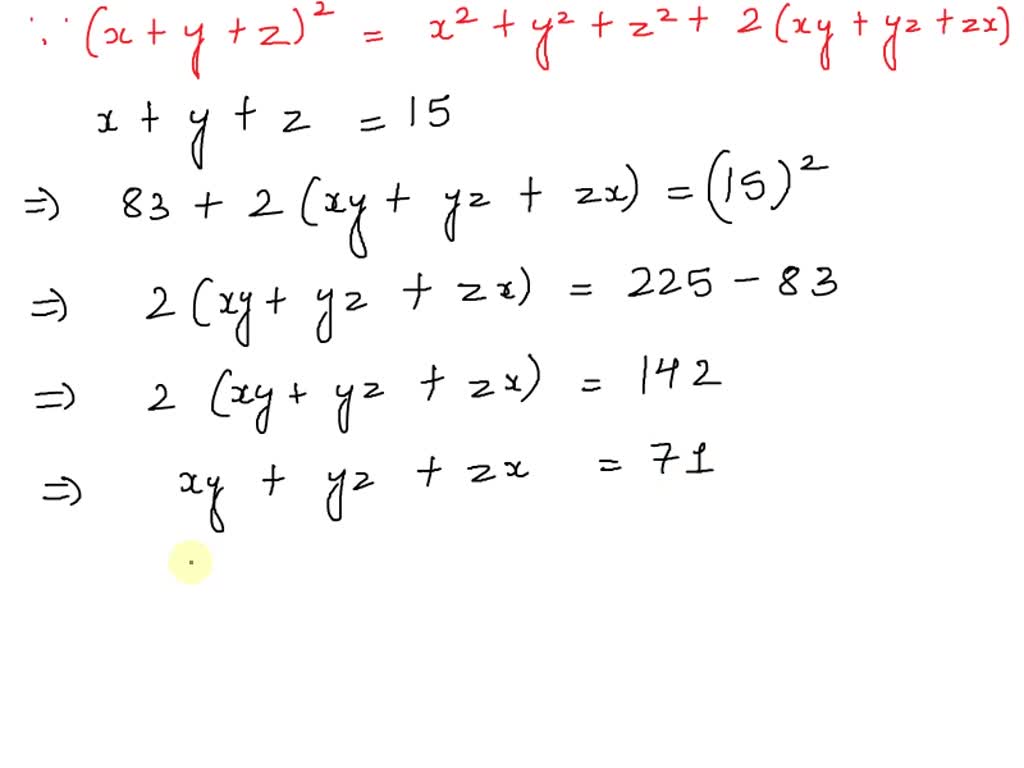



Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15
Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by Final result 3xyz • (x2y x2z xy2 xz2 y2z yz2) Step by step solution Step 1 Equation at the end of step 1 ( ( (x3)• ( (yz)3)) ( (y3)• ( (zx)3)))z3• (xy)3 Step 2 Find 𝑑𝑖𝑣 𝐹⃗ and 𝑐𝑢𝑟𝑙 𝐹⃗, where \overrightarrow {F}=\bigtriangledown (x^ {3}y^ {3}z^ {3}3xyz) F = (x3y3 z3 −3xyz) , , , Find the directional derivative of a given equation at the point




Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z Z X 2
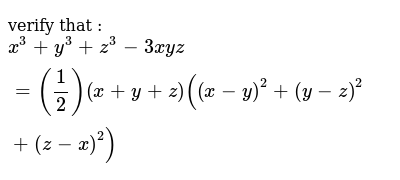



Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2
X y z = 2 and xy yz zx = 11, then the value of x 3 y 3 z 3 3xyz is 1 71 2 74 3 69 4 78 quantitativeaptitude;Factorize x^3 y^3 z^3 = 3xyz(xyz)^3 (x y z) (x y z) (x y z) We multiply using the FOIL Method x * x = x^2 x * y = xy x * z = xz y * x = xy




Polynomials Ppt Video Online Download




If X Y Z 6 And Xy Yz Zx 11 Then What Is The Value Of X 3 Y 3 Z 3 3xyz
Solved Factorize the polynomial x^3y^3z^3−3xyz Solve your problem for the price of one coffee Available 24/7 Math expert for every subjectThe equation x^3 37 y^3 = 1 has aX y z = 8, xy yz zx = 15 Formula used x 3 y 3 z 3 3xyz = (x y z) (x 2 y 2 z 2 xy yz zx) (x y z) 2 = x 2 y 2 z 2 2(xy yz zx) x 3 y 3 z 3 3xyz = (x y z)



X 3 Y 3 Formula
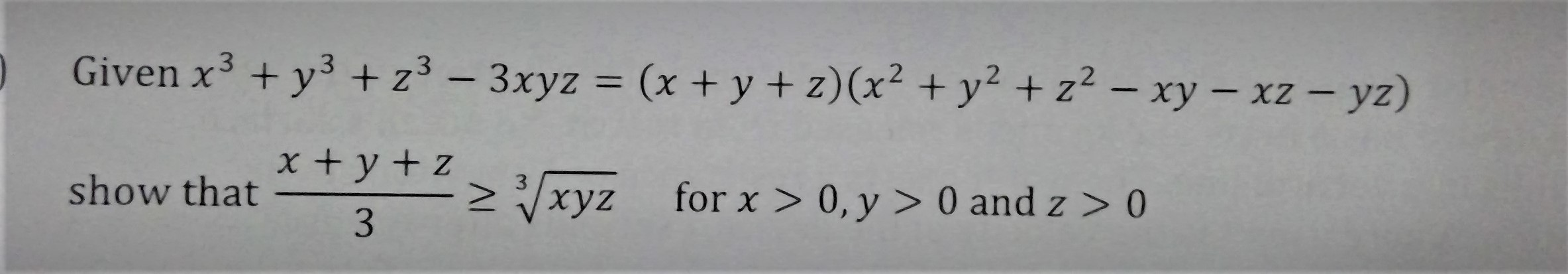



Solved 0 Given X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Chegg Com
Algebra Factor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2)$\Rightarrow {{x}^{3}}{{y}^{3}}{{z}^{3}}3xyz=\left( 12 \right)\left( 33 \right)$ Multiplying the equation, we get \ \Rightarrow {x^3} {y^3} {z^3} 3xyz = 396\Algebra Factor x^3y^3z^3 x3y3 z3 x 3 y 3 z 3 Rewrite x3y3 x 3 y 3 as (xy)3 ( x y) 3 (xy)3 z3 ( x y) 3 z 3 Since both terms are perfect cubes, factor using the sum of cubes formula,




Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15




Factorize X3 Y3 Z3 3xyz Brainly In
Now the formula x 3 y 3 z 3 – 3xyz = (x y z) (x 2 y 2 z 2 – xy – yz – zx) Putting the given values ⇒ 80 – 3xyz = 8 × (24 – ) ⇒ 3xyz = 80 – 32 = 48 ⇒ xyz = 16 ∴ Required valueX = 551, y = 552 and z = 557 we know the alternate way to write the forFactoring Calculator Online calculator factors single variable or multivariable polynomial with step by step explanations Start by entering your expression in the formula pane below Example x 4




Maths Formula For Cbse Class 10 Math Formula Cbse Class 10 Cbse Math




Class 9 Exercise 2 If X 3 Y 4 And Z 7 Then X3 Y3 Z3 3xyz Is Equal To
If x = 551, y = 552 and z = 557, then what is the value of x 3 y 3 z 3 – 3xyz? Also, the equation of the form x^3 d y^3 = 1 has a solution for which y= 3 if and only if d = u(27 u^2 9 u 1) for some integer u, for example;You can put this solution on YOUR website!




New Solutions Of 1 Download Table
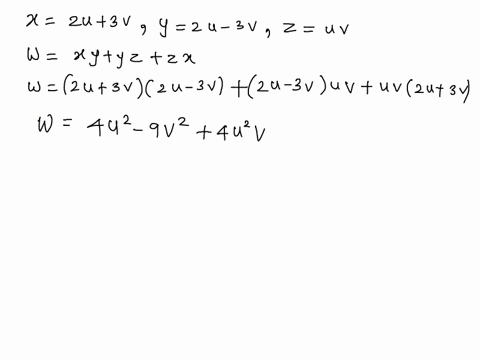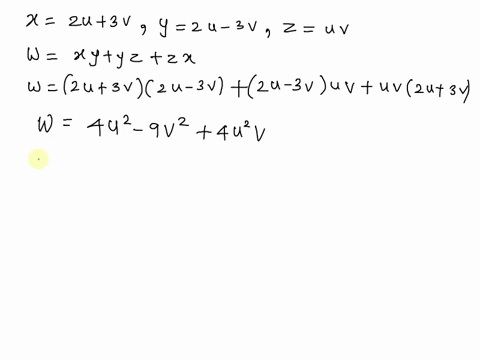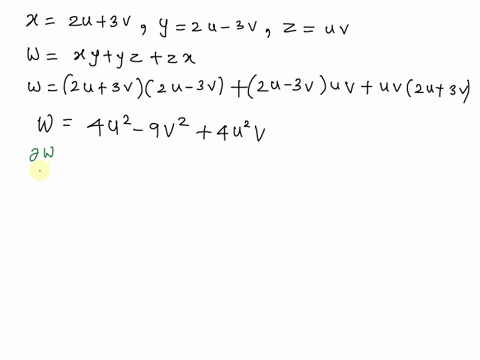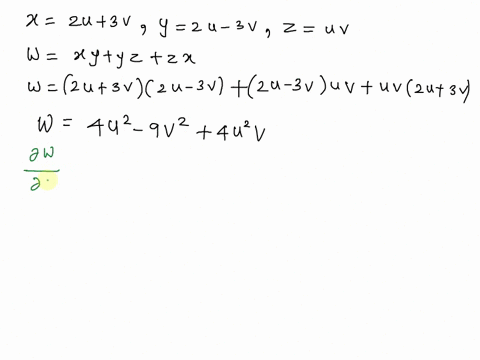



Solved For The Functions W Xy Yz Xz X Zu 3v Y Zu 3v And Z Uv Express And Using The Chain Rule And By Expressing Directly In Terms Of
A) 36 b) 40 c) 42 d) 48 The polynomial is (a) homogeneous and (b) symmetric in x, y, z Good review of those properties So it factors as (x y z)(Ax2 Ay2 Az2 Bxy Byz Bxz) Then let x = 0 and we We can write this as x^3 y^3 z^3 – 3xyz = (x y z)(x^2 y^2 z^2 – xy – yz – za) Using the formula (x y z)^2 = x^2 y^2 z^2 2(xy yz zx) Substituting the values



Q Tbn And9gcqm Sck6bfmyzpj2bmaosgzrrxmcqdgwf2teaddjkcwtgd1z6vlj Cg Usqp Cau




If X Y Z 0 Then Prove That X3 Y3 Z3 3xyz Youtube
If x y z = 8, xy yz zx = 15 then find x 3 y 3 z 3 3xyz 1 151 2 152 3 153 4 251 5 180 quantitativeaptitude;




Rd Sharma Solutions For Class 7 Maths Chapter 7 Algebraic Expressions Download Free Pdf
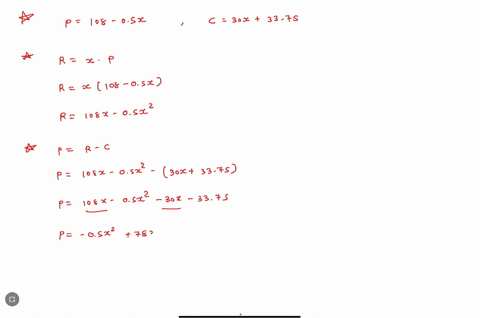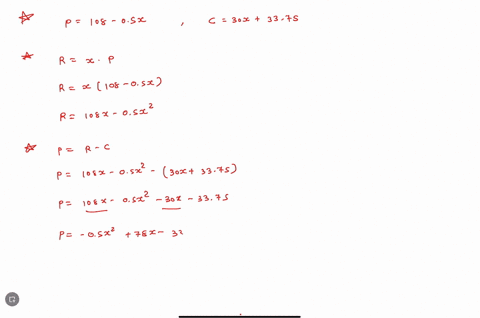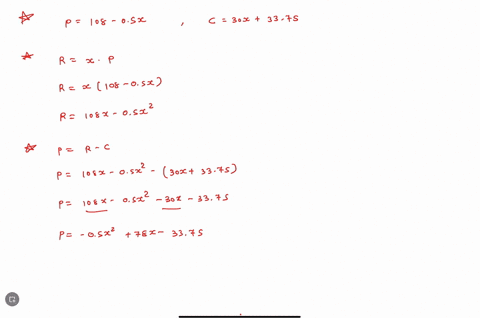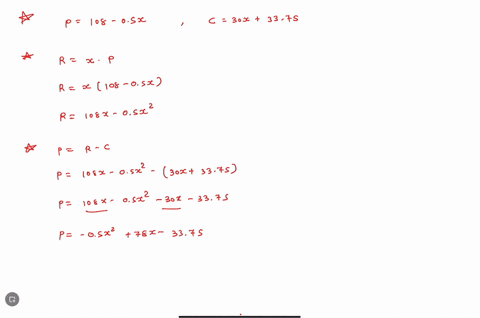



Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15



Cbse 9 Math Cbse Polynomials Ncert Solutions




Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes



Cbse 9 Math Cbse Polynomials Ncert Solutions




Productos Notables Formulas Pdf




Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Brainly In




Factorise 27x 3 Y 3 Z 3 9xyz Using Identity




Polynomials Ppt Video Online Download



If X3 Y3 Z3 6xyz And Xyz X Y Z What Is The Value Of X Y 2 Y Z 2 Z X 2 Quora



Verify Euler S Theorem For The Function U X 3 Y 3 3xy 2 Sarthaks Econnect Largest Online Education Community




Foliation Of The Real Projective Plane By The Family Of Elliptic Download Scientific Diagram
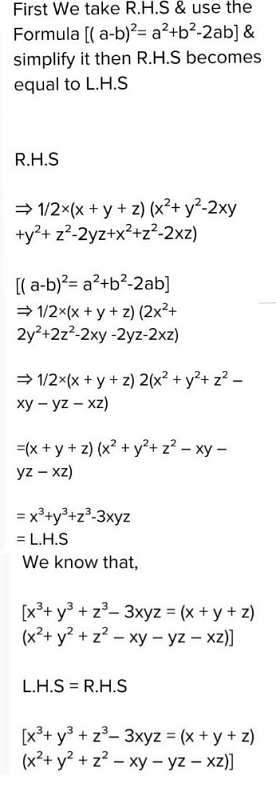



Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Can Any One Solve This Question Edurev Class 9 Question
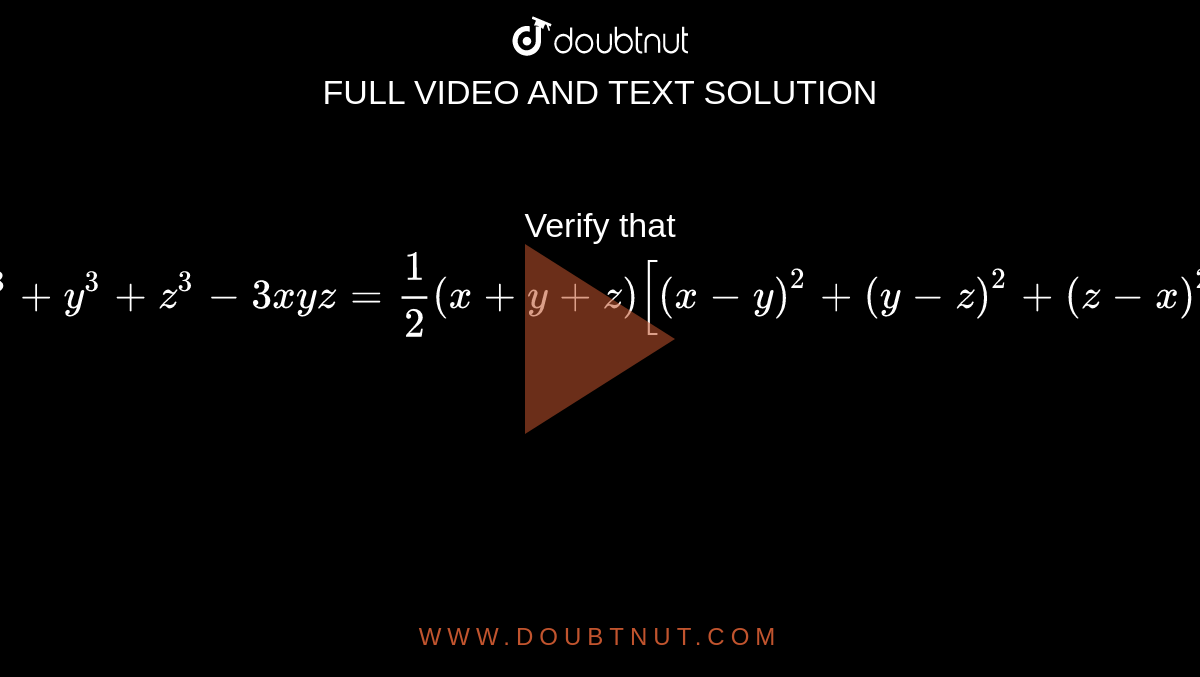



Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2




Ex 2 5 12 Verify That X3 Y3 Z3 3xyz 1 2 Ex 2 5




If X Y Z 0 Show That X 3 Y 3 Z 3 3xyz With Video




Pdf On Solving The Diophantine Equation 𝑥 𝑦 𝑧 𝑘 On A Vector Computer




Please Solve The Assignment Attached Find 6 Answers Solutions Learnpick Resources
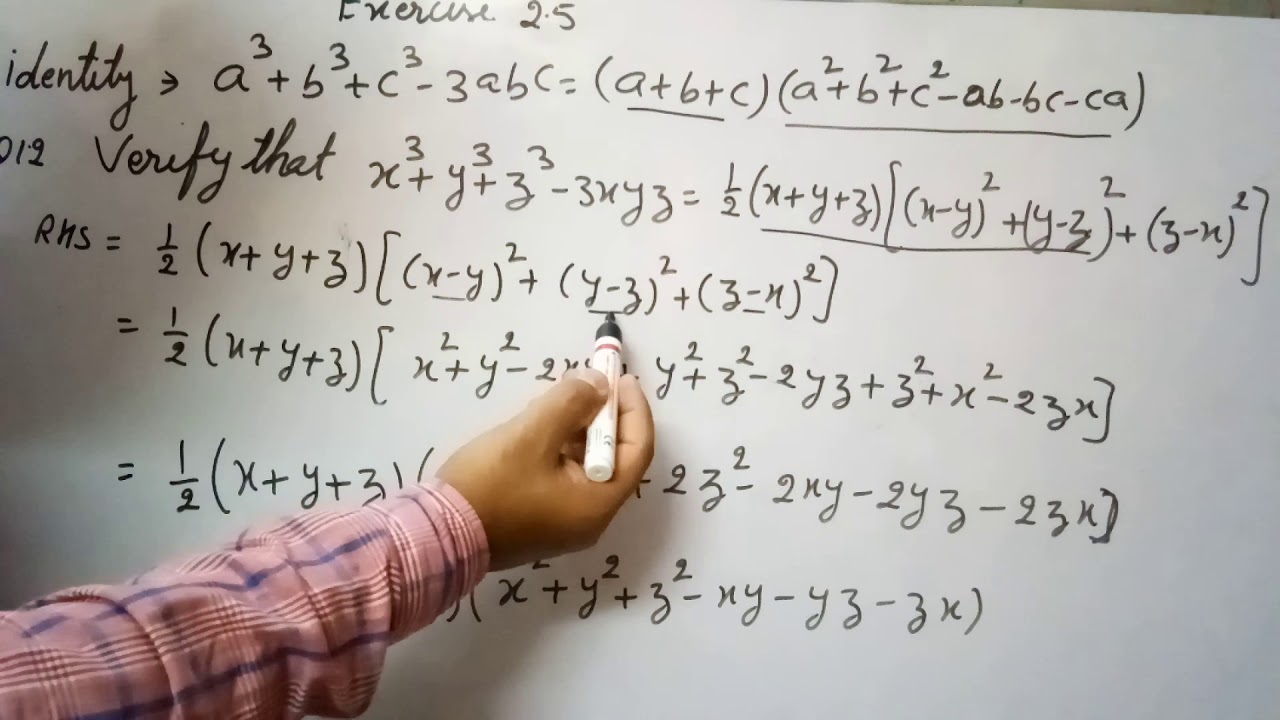



Ex 2 5 Q No 12 Verify X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Youtube



Q Tbn And9gcqipaz24deyu9ejnwo4ogprejdlr4t66vaq4p4akyl8jytzulnvntf1 Usqp Cau




Using The Identity And Proof X 3 Y 3 Z 3 3xyz X Y Z X 2 Y 2 Z 2 Xy Yz Zx




Find The Value Of X 3 Y 3 Z 3 3xyz If X 2 Y 2 Z 2 And X Y Z 15 Youtube




Prove That X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Brainly In




Unit 2
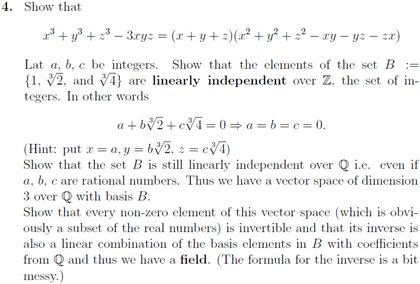



Show That X3 Y3 Z3 3xyz X Y Z X2 Y2 Chegg Com



Taller De Algebra




Polynomials Ppt Video Online Download
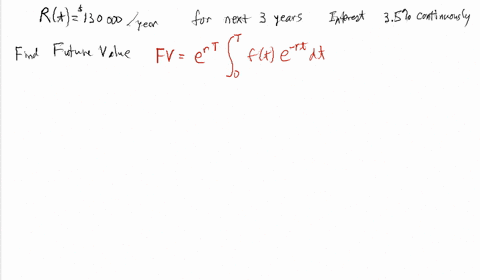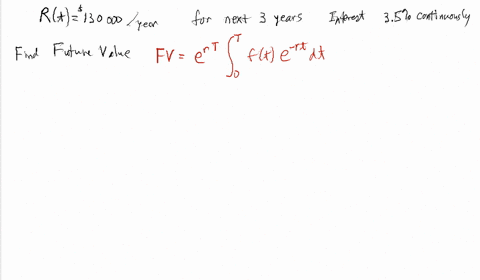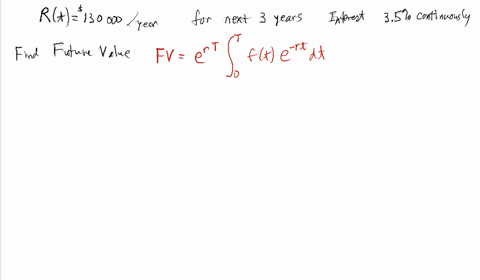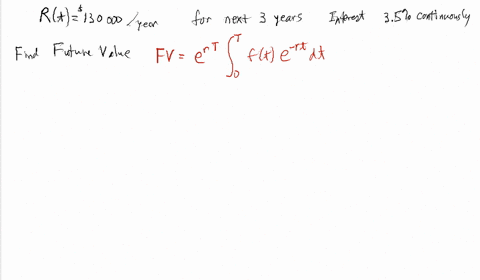



Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15



If X Z 225 And Y 226 Then What Is The Value Of X Y Z 3xyz Quora




How To Factorise Using The Identity X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Youtube



因式分解 X 3 Y 3 Z 3 3xyz 紀算補習班 數學補習班 三重 文理補習班 國小數學 國中數學 隨意窩xuite日誌




Determinants Class 12 Maths Important Questions Chapter 4 Learn Cbse
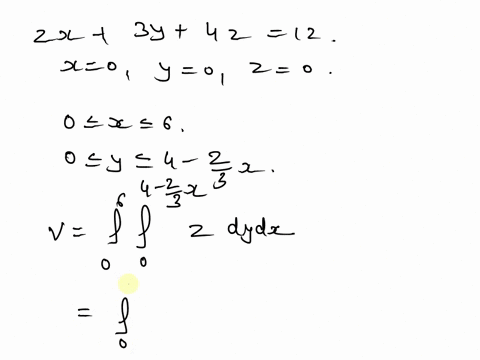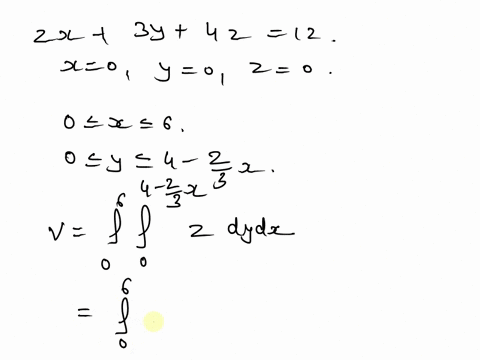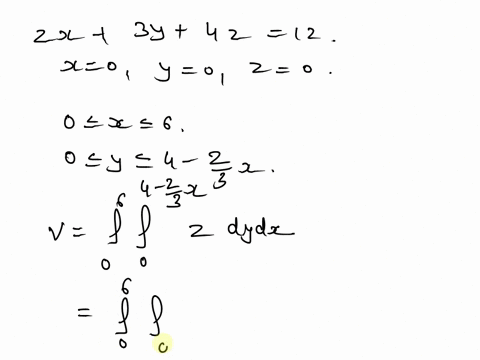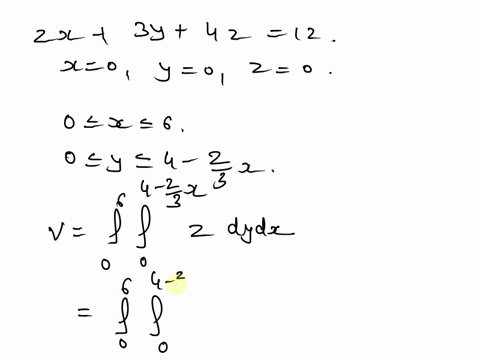



Solved Find The Value Of X3 Y3 Z3 3xyz If X2 Y2 Z2 X Y Z 15



Using Identities Prove That X 3 Y 3 Z 3 Or Equal To 3xyz Where X Y Z Are Positive Real Numbers Quora
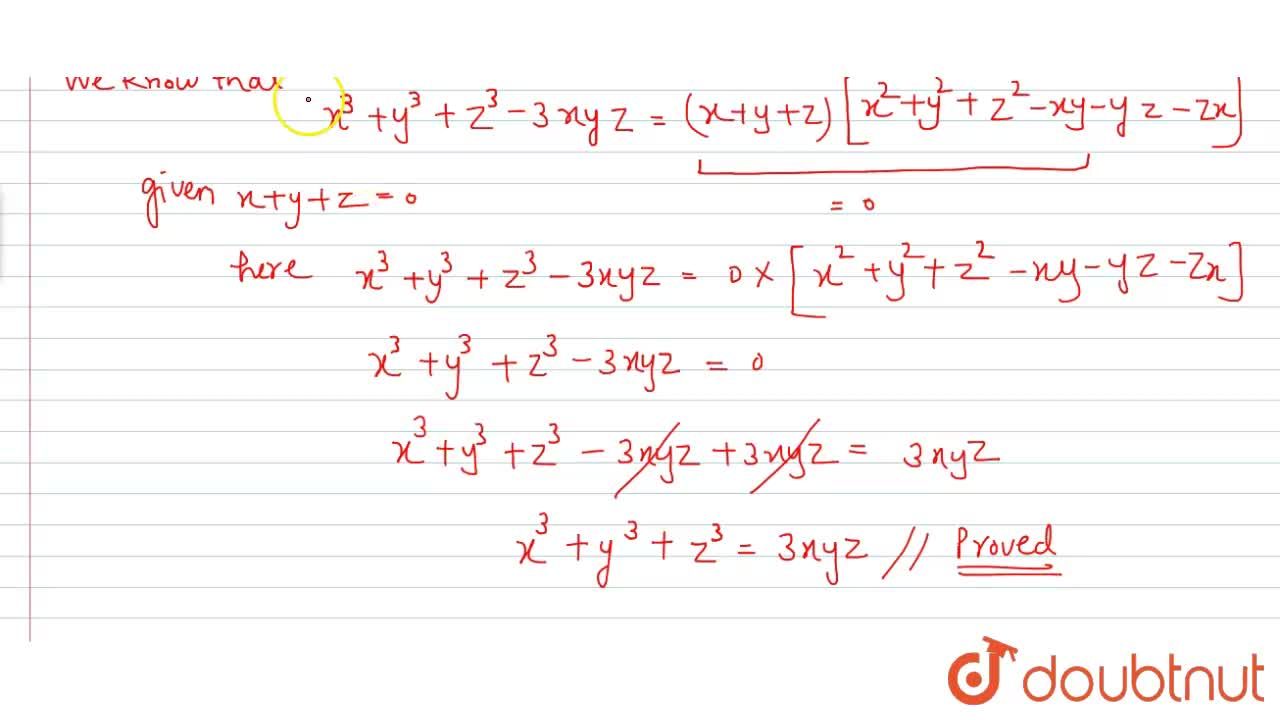



If X Y Z 0 Show That X 3 Y 3 Z 3 3x Y Z




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




What Is The Formula Of X3 Y3 Z3 Book Vea




Class 9 Exercise 2 If X 3 Y 4 And Z 7 Then X3 Y3 Z3 3xyz Is Equal To




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes




If X Y Z 0 Then The Value Of X3 Y3 Z3 Is




Icse Class 10 Answered




Showimage Php Formula D7cce7fd28d9cde1981b9fe2b1ed4448 Png D 0



2




Evaluate X 3 Y 3 Z 3 3xyz When X 2 Y 1 And Z 3
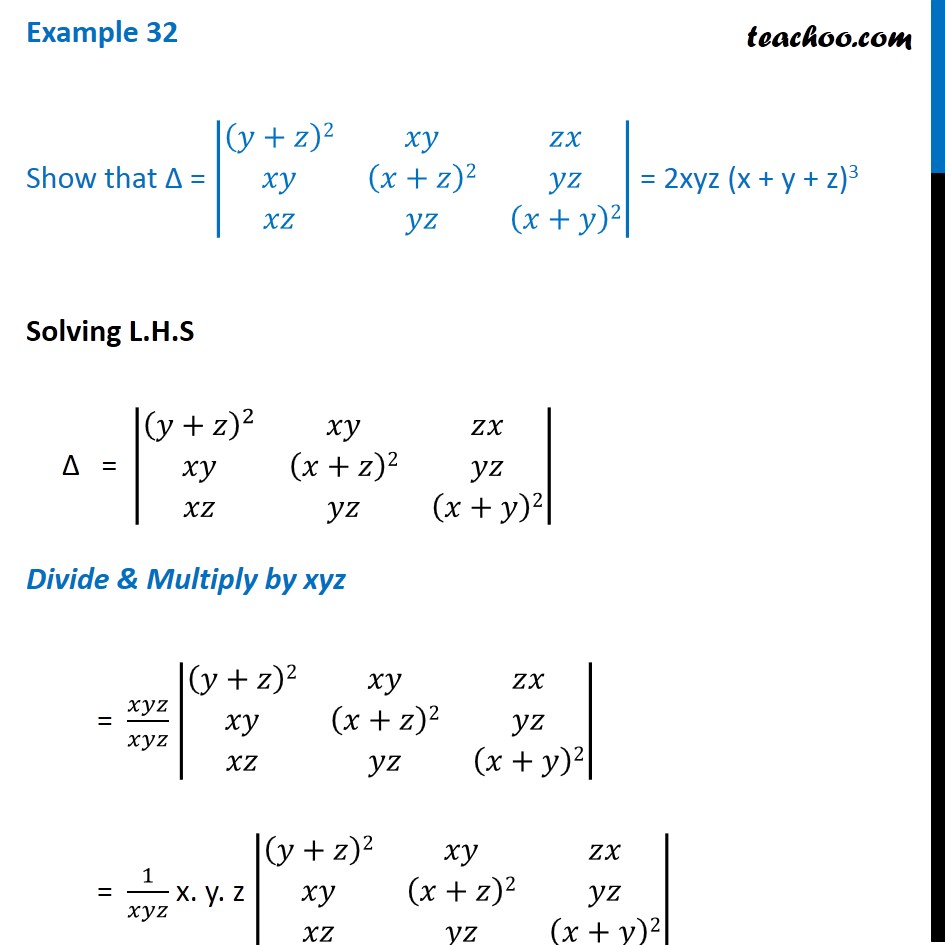



Example 32 Show That Determinant 2xyz X Y Z 3 Class 12




2 Polynomials Note Blast



How To Divide X 3 Y 3 Z 3 3xyz By X Y Z Quora




If X Y Z 0 Show That X 3 Y 3 Z 3 3xyz With Video




Ex 2 5 14 Without Calculating Find 28 3 15 3 13 3




Find The Cube Root Of X3 Y3 Z3 Brainly In




Unit 2




Ncert Solutions For Class 9 Maths Exercise 2 5 22 23




X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Proof It Lhs To Rhs Brainly In




Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Brainly In




Verify That X3 Y3 Z3 3xyz Half Of X Y Z X Y 2 Y Z 2 Z X 2 Maths Polynomials Meritnation Com




Pdf On Solving The Diophantine Equation 𝑥 𝑦 𝑧 𝑘 On A Vector Computer



Verify Euler S Theorem For The Function U X 3 Y 3 3xy 2 Sarthaks Econnect Largest Online Education Community




Factoring Formulas In Algebra What Are Factoring Formulas
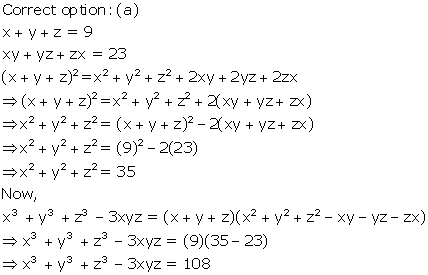



R S Aggarwal And V Aggarwal Solutions For Class Maths Cbse Chapter 3 Factorisation Of Polynomials Topperlearning




Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Brainly In




Bits Of Math Problem Cubes And Cube Roots




2 Two Cube 5 Five Cube 10 Ten Cube Pdf Free Download




Prove That X Y3 Y Z3 Z X3 3x Yy Zz X 2x3 Y3 Z3 3xyz Maths Polynomials Meritnation Com




Polynomials Ppt Video Online Download




Pdf On Some Properties Of Xi Yi Zi Dxyz X3 Y3 Z3 Dxyz And X6 Y6 Z6 Dxyz And A Critique Of Solutions For The Markoff Equation X2 Y2 Z2 Axyz



Cbse 9 Math Cbse Polynomials Ncert Solutions




Ex 2 5 11 Factorise 27 X3 Y3 Z3 9xyz Class 9 Ex 2 5
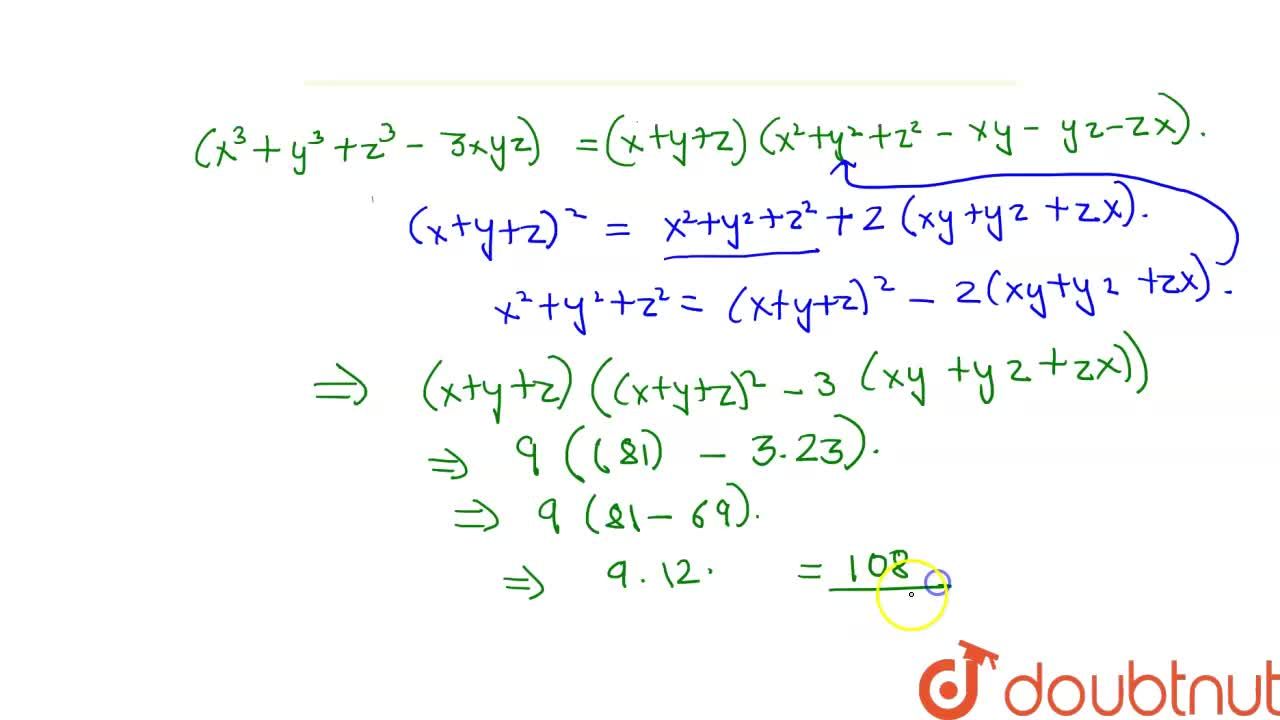



If X Y Z 9 And Xy Yz Zx 23 The Value Of X 3 Y 3 Z 3 3xyz




If X Y Z Is Equal To Zero Show That X Cube Y Cube Z Cube Is Equals To 3 X Y Z Brainly In




Pdf On Solving The Diophantine Equation 𝑥 𝑦 𝑧 𝑘 On A Vector Computer




Solving A Quadratic Equation By Factoring A Plus Topper




Rd Sharma Ex 5 4 Chapter 5 Class 9 Factorization Of Algebraic Expressions Solutions



If X Y Z 6 And Xy Yz Zx 10 Then What Is The Solution Of X 3 Y 3 3xyz Quora



0 件のコメント:
コメントを投稿